
The website of Vedantu is very useful as it provides the students with answers to all their academic questions. The resources available at Vedantu are 100% reliable and accurate which helps the students to learn and practice while being satisfied from the user end.
#RANK OF A MATRIX FREE#
Vedantu is India’s leading online platform that provides students with all the necessary study material free of cost. Now, the above matrix is in echelon form.
#RANK OF A MATRIX FULL#
When the rank equals the smallest dimension it is called the full rank matrix. So, the rank of the zero matrices is zero. Hence it has an independent row (or column). Rank linear algebra refers to finding column rank or row rank collectively known as the rank of the matrix.

Rank + Nullity is the number of all columns in matrix A. In other words, it can be defined as the dimension of the null space of matrix A called the nullity of A. The nullity of a matrix is defined as the number of vectors present in the null space of a given matrix. The rank of a matrix cannot exceed more than the number of its rows or columns.

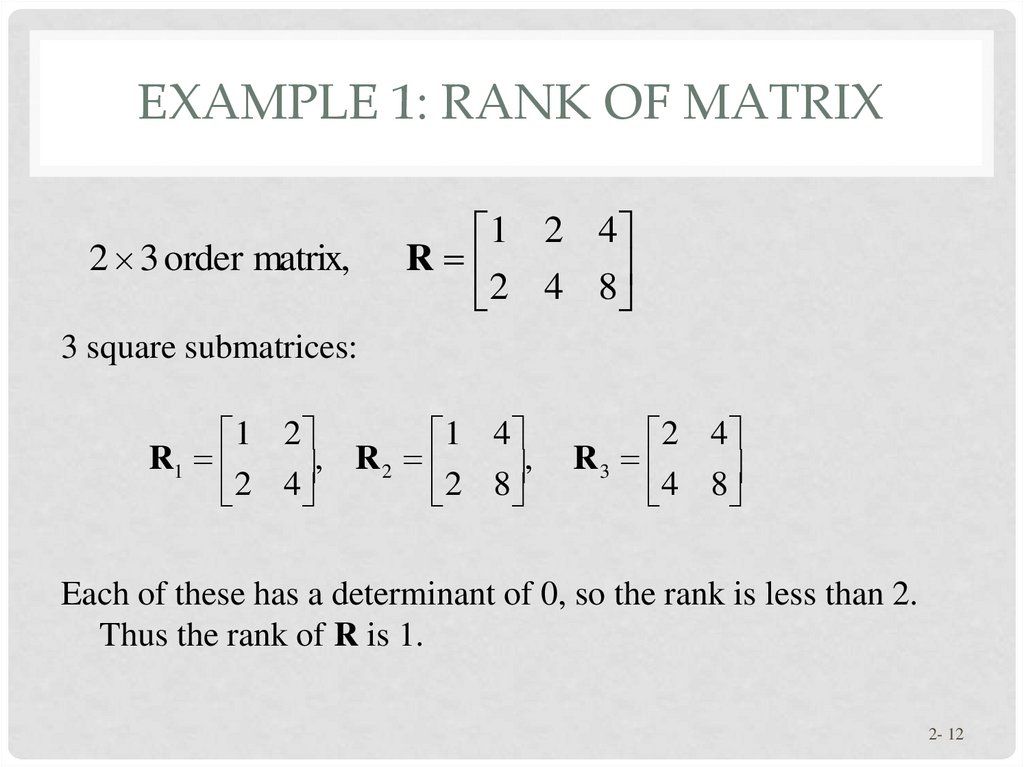
The rank of the matrix is the dimension of the vector space obtained by its columns. A matrix is said to be of rank zero when all of its elements become zero. ρ(A) is used to denote the rank of matrix A. The rank of the matrix refers to the number of linearly independent rows or columns in the matrix. Minor of the matrix is the determinant of the square matrix that is obtained by deleting one row and one column from some larger square matrix.įor the students of Class 12 studying subjects like Maths, Matrix is an important concept as it forms the base for more detailed concepts later, the topic is one of the most crucial from the perspective of the JEE main exam, and thus, it is imperative for the students to wisely learn the same. A matrix (Plural is matrices) is a rectangular array of numbers, symbols, or expressions, which are arranged in the form of rows and columns. Matrix obtained by deleting some rows and some columns of matrix A is known as the sub-matrix of A. To define the rank of a matrix, we should have prior knowledge of sub-matrices and minors of a matrix.


 0 kommentar(er)
0 kommentar(er)
